Difference Of Two Convergent Series

Mathematical series with a finite sum

In mathematics, a series is the sum of the terms of an infinite sequence of numbers. More than precisely, an space sequence ${\displaystyle (a_{0},a_{i},a_{2},\ldots )}$ defines a series S that is denoted

${\displaystyle Southward=a_{0}+a_{1}+a_{2}+\cdots =\sum _{k=0}^{\infty }a_{m}.}$

The n thursday partial sum S _{due north} is the sum of the first due north terms of the sequence; that is,

${\displaystyle S_{n}=\sum _{k=1}^{n}a_{chiliad}.}$

A serial is convergent (or converges) if the sequence ${\displaystyle (S_{1},S_{two},S_{3},\dots )}$ of its partial sums tends to a limit; that means that, when adding one ${\displaystyle a_{k}}$ after the other in the order given past the indices, i gets partial sums that get closer and closer to a given number. More precisely, a series converges, if there exists a number ${\displaystyle \ell }$ such that for every arbitrarily pocket-size positive number ${\displaystyle \varepsilon }$ , there is a (sufficiently large) integer ${\displaystyle North}$ such that for all ${\displaystyle due north\geq N}$ ,

${\displaystyle \left|S_{n}-\ell \right|<\varepsilon .}$

If the series is convergent, the (necessarily unique) number ${\displaystyle \ell }$ is called the sum of the series.

The same annotation

${\displaystyle \sum _{grand=i}^{\infty }a_{m}}$

is used for the series, and, if it is convergent, to its sum. This convention is similar to that which is used for addition: a + b denotes the operation of adding a and b also as the effect of this addition, which is chosen the sum of a and b.

Any series that is not convergent is said to be divergent or to diverge.

Examples of convergent and divergent series [edit]

Convergence tests [edit]

At that place are a number of methods of determining whether a series converges or diverges.

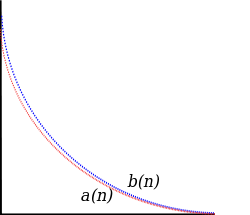
If the blue series, ${\displaystyle \Sigma b_{n}}$ , can exist proven to converge, then the smaller series, ${\displaystyle \Sigma a_{northward}}$ must converge. By contraposition, if the red series ${\displaystyle \Sigma a_{northward}}$ is proven to diverge, then ${\displaystyle \Sigma b_{n}}$ must likewise diverge.

Comparing examination. The terms of the sequence ${\displaystyle \left\{a_{due north}\correct\}}$ are compared to those of some other sequence ${\displaystyle \left\{b_{northward}\right\}}$ . If, for all north, ${\displaystyle 0\leq \ a_{northward}\leq \ b_{n}}$ , and ${\textstyle \sum _{n=1}^{\infty }b_{northward}}$ converges, then and so does ${\textstyle \sum _{n=ane}^{\infty }a_{northward}.}$

All the same, if, for all n, ${\displaystyle 0\leq \ b_{due north}\leq \ a_{n}}$ , and ${\textstyle \sum _{n=i}^{\infty }b_{n}}$ diverges, and then and then does ${\textstyle \sum _{n=1}^{\infty }a_{n}.}$

Ratio examination. Presume that for all n, ${\displaystyle a_{n}}$ is non nix. Suppose that at that place exists ${\displaystyle r}$ such that

${\displaystyle \lim _{due north\to \infty }\left|{\frac {a_{northward+ane}}{a_{n}}}\right|=r.}$

If r < i, then the series is admittedly convergent. If r > 1, then the series diverges. If r = 1, the ratio test is inconclusive, and the series may converge or diverge.

Root test or due northth root examination. Suppose that the terms of the sequence in question are not-negative. Define r as follows:

${\displaystyle r=\limsup _{n\to \infty }{\sqrt[{n}]{|a_{n}|}},}$

where "lim sup" denotes the limit superior (possibly ∞; if the limit exists it is the aforementioned value).

If r < i, and so the serial converges. If r > i, and so the series diverges. If r = 1, the root examination is inconclusive, and the series may converge or diverge.

The ratio test and the root exam are both based on comparing with a geometric serial, and as such they work in similar situations. In fact, if the ratio test works (pregnant that the limit exists and is not equal to i) and so and then does the root test; the antipodal, however, is not true. The root examination is therefore more than generally applicable, simply as a applied affair the limit is often hard to compute for commonly seen types of serial.

Integral test. The series can be compared to an integral to establish convergence or departure. Let ${\displaystyle f(north)=a_{north}}$ be a positive and monotonically decreasing function. If

${\displaystyle \int _{i}^{\infty }f(ten)\,dx=\lim _{t\to \infty }\int _{1}^{t}f(ten)\,dx<\infty ,}$

then the serial converges. But if the integral diverges, then the series does and so likewise.

Limit comparing test. If ${\displaystyle \left\{a_{due north}\correct\},\left\{b_{due north}\right\}>0}$ , and the limit ${\displaystyle \lim _{n\to \infty }{\frac {a_{n}}{b_{northward}}}}$ exists and is not zero, then ${\textstyle \sum _{north=i}^{\infty }a_{n}}$ converges if and just if ${\textstyle \sum _{n=1}^{\infty }b_{north}}$ converges.

Alternating series test. Likewise known every bit the Leibniz criterion, the alternating series test states that for an alternating serial of the course ${\textstyle \sum _{n=i}^{\infty }a_{n}(-ane)^{due north}}$ , if ${\displaystyle \left\{a_{n}\right\}}$ is monotonically decreasing, and has a limit of 0 at infinity, so the series converges.

Cauchy condensation test. If ${\displaystyle \left\{a_{due north}\correct\}}$ is a positive monotone decreasing sequence, so ${\textstyle \sum _{n=1}^{\infty }a_{n}}$ converges if and only if ${\textstyle \sum _{yard=i}^{\infty }2^{one thousand}a_{ii^{k}}}$ converges.

Dirichlet's test

Abel's exam

Provisional and absolute convergence [edit]

For whatever sequence ${\displaystyle \left\{a_{1},\ a_{ii},\ a_{3},\dots \right\}}$ , ${\displaystyle a_{north}\leq \left|a_{n}\right|}$ for all due north. Therefore,

${\displaystyle \sum _{n=1}^{\infty }a_{n}\leq \sum _{n=1}^{\infty }\left|a_{n}\correct|.}$

This means that if ${\textstyle \sum _{n=i}^{\infty }\left|a_{n}\right|}$ converges, then ${\textstyle \sum _{n=i}^{\infty }a_{n}}$ also converges (merely not vice versa).

If the series ${\textstyle \sum _{n=1}^{\infty }\left|a_{n}\right|}$ converges, so the series ${\textstyle \sum _{north=1}^{\infty }a_{due north}}$ is admittedly convergent. The Maclaurin series of the exponential function is absolutely convergent for every complex value of the variable.

If the serial ${\textstyle \sum _{n=1}^{\infty }a_{n}}$ converges but the series ${\textstyle \sum _{n=1}^{\infty }\left|a_{northward}\right|}$ diverges, so the series ${\textstyle \sum _{n=i}^{\infty }a_{n}}$ is conditionally convergent. The Maclaurin series of the logarithm function ${\displaystyle \ln(1+ten)}$ is conditionally convergent for x = 1.

The Riemann series theorem states that if a series converges conditionally, it is possible to rearrange the terms of the series in such a fashion that the serial converges to any value, or fifty-fifty diverges.

Uniform convergence [edit]

Let ${\displaystyle \left\{f_{1},\ f_{2},\ f_{3},\dots \right\}}$ be a sequence of functions. The serial ${\textstyle \sum _{north=1}^{\infty }f_{north}}$ is said to converge uniformly to f if the sequence ${\displaystyle \{s_{north}\}}$ of partial sums defined past

${\displaystyle s_{n}(ten)=\sum _{m=ane}^{north}f_{k}(10)}$

converges uniformly to f.

There is an counterpart of the comparison exam for space series of functions called the Weierstrass M-exam.

Cauchy convergence criterion [edit]

The Cauchy convergence criterion states that a serial

${\displaystyle \sum _{n=1}^{\infty }a_{n}}$

converges if and simply if the sequence of partial sums is a Cauchy sequence. This ways that for every ${\displaystyle \varepsilon >0,}$ in that location is a positive integer ${\displaystyle North}$ such that for all ${\displaystyle north\geq g\geq N}$ nosotros have

${\displaystyle \left|\sum _{k=k}^{n}a_{chiliad}\right|<\varepsilon ,}$

which is equivalent to

${\displaystyle \lim _{due north\to \infty \atop thousand\to \infty }\sum _{one thousand=due north}^{north+thousand}a_{k}=0.}$

See also [edit]

• Normal convergence
• List of mathematical series

External links [edit]

• "Series", Encyclopedia of Mathematics, Ems Press, 2001 [1994]
• Weisstein, Eric (2005). Riemann Series Theorem. Retrieved May 16, 2005.

Difference Of Two Convergent Series,

Source: https://en.wikipedia.org/wiki/Convergent_series

Posted by: crewsmistne.blogspot.com

Share this post